Hay varias familias de politopos simétricos[1] con simetría irreducible que tienen un miembro en más de una dimensionalidad. Aquí se tabulan con los gráficos de proyección de sus polígonos de Petrie y con sus diagramas de Coxeter-Dynkin:
| Tabla de familias de politopos irreducibles | ||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Familia n |
n-símplex | n-hipercubo | n-politopo de cruce | n-demicubo | 1k2 | 2k1 | k21 | Politopo pentagonal | ||||||||
| Grupo | An | Bn |
|
|
Hn | |||||||||||
| 2 |  |
 |
 p-gono (ejemplo: p=7) |
 Hexágono |
 Pentágono | |||||||||||
| 3 |  Tetraedro |
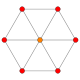 Cubo |
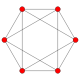 Octaedro |
 Tetraedro |
 Dodecaedro |
 Icosaedro | ||||||||||
| 4 |  Pentácoron |
 |
 Hexadecacoron |
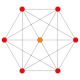 |
 Icositetracoron |
 Hecatonicosacoron |
 Hexacosicoron | |||||||||
| 5 |  5-símplex |
 Penteracto |
 5-ortoplex |
 5-demicubo |
||||||||||||
| 6 |  6-símplex |
 Hexeracto |
 6-ortoplex |
 6-demicubo |
 122 |
 221 |
||||||||||
| 7 |  7-símplex |
 Hepteracto |
 7-ortoplex |
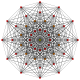 7-demicubo |
 132 |
 231 |
 321 |
|||||||||
| 8 |  8-símplex |
 Octoracto |
 8-ortoplex |
 8-demicubo |
 142 |
 241 |
 421 |
|||||||||
| 9 |  9-símplex |
 Eneracto |
 9-ortoplex |
 9-demicubo |
||||||||||||
| 10 |  10-símplex |
 decaracto |
 10-ortoplex |
 10-demicubo |
||||||||||||
Referencias
- ↑ Valery V. Volchkov, Vitaly V. Volchkov (2013). Offbeat Integral Geometry on Symmetric Spaces. Springer Science & Business Media. pp. 364 de 592. ISBN 9783034805728. Consultado el 28 de abril de 2023.








